LANGUAGE
The Beautiful Formula Language allows to create new compositions and to notate already existing paintings.
We are constantly working on the improvement of The Beautiful Formula Language. Please, feel free to send us your suggestion, comment or any kind of question.
thebeautifulformula@googlemail.com
The Beautiful Formula Collective
SIGNS AND SYMBOLS
U UNIT
M METER
A AREA
T TAKT
R RHYTHMICAL MOTIVE
E ELEMENT
P PROCEDURE
# ENTRY
* hits the meter
[] within the same area
#(n) number of entries
∞ number of entries flexible
(n) size of unit
≈ size of the unit is corresponding for all elements
\ bigger than
/ less than
|| order fixed
> n occupy n
-> go to
{ n out of n possible
+ has to touch
_ has not to touch
≠ not
V vertical
H horizontal
F flexibel
ⁿ ordinal number
L line
{ n } polygon with n edges
U UNIT
The unit defines the basic size of any kind of mark on the surface. 1 is the smallest size of a mark, 2 is twice as big, 3 is three times bigger and so forth…
Examples: 1,2,3
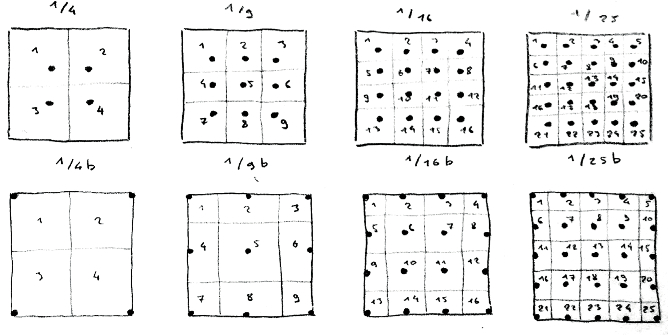
M METER
The meter is a fixed point with a surrounding area.
The meter is used to accomplish an equal partition of the surface.
A meter is numbered from left to right and top to bottom.
Examples: 1/4, 1/9, 1/16, 1/25
Meters with the additional letter b include the surface border and the four corners.
Examples: 1/4b, 1/9b, 1/16b, 1/25b
A AREA
The area surrounds the meter and is defined by the rows and columns between meters. The area and its meter have the same numbering.
Examples: 1/4, 1/9, 1/16, 1/25 & 1/4b, 1/9b, 1/16b, 1/25b
T TAKT
The takt is a fixed number of units during one single entry.
Examples: takt 2, 3, 4 & 5 (A T2, B T3, C T4, D T5)
R RHYTHMICAL MOTIVE
The rhythmical motive is a fixed sequence of units during one single entry.
Examples: 2,2,3,1 or 1,2,1,3,1,5
E ELEMENT
The element is a unique character of a unit.
Elements are named with a, b, c …
Examples:
M 1/4
R 2,2,3,1
E a,b,c,d
P PROCEDURE
The procedure defines the painting process.
Examples:
M 1/4
R 1,2,1,3,1,5
E a,b,c,d,e,f
P *a1,b2,c1,d3,e1,f5
# ENTRY
The entry defines how often each element can participate.
Examples:
M 1/4
R 1,2,1,3,1,5
E a,b,c,d,e,f
P *a1,b2,c1,d3,e1,f5 #3
* hits the meter
M 1/4
R 2,2,3,1
E a,b,c,d
P *a2,*b2,*c3,*d1
[] within the same area
M 1/4
R 2,2,3,1
E a,b,c,d
P
[*a2,b2,c3,d1]
[*b2,c2,d3,a1]
[*c2,d2,a3,b1]
[*d2,a2,b3,c1]
#(n) number of entries
M 1/9
R 2,2,3,1
E a,b,c,d
P
[*a] #(1)
[*b] #(2)
[*c] #(2)
[*d] #(4
∞ number of entries flexible
M 1/4
R 2,2,3,1
E a,b
P
a #∞
b M4 [*2,2,3,1] #1
(n) size of unit
M 1/4
R –,–,–,–,– (1-5) (size of unit can vary from 1-5)
E a
P [*a] > 1{M1/4
≈ size of the unit is corresponding for all elements
M 1/4
A 1/4
R 2,2,3,1
E a,b,c,d ≈
P
a A1[*2,2,3,1]
b A2[*2,2,3,1]
c A3[*2,2,3,1]
d A4[*2,2,3,1]
\ bigger than
A 1/4
E a
P [*-\(3),2,2,3,1] > 1{A1/4
/ less than
A 1/4
E a
P [*-/(1),2,2,3,1] > 1{A1/4
|| order fixed
M 1/4
R 1,1,1,2,3
E |a,b,c,d| (the entry of the elements has to be in the same order)
P
M1 [*a]
M4 [*a,b,c,d]
> n occupy n
M 1/4, 1/9
R 2,2,3,1
E a,b
P
M 1/4 [*a] > M4
M 1/9 [*b] > M5
{ n out of n possible
M 1/4
R 2,2,3,1
E a,b
P
[*a]
[*a]
[*a]
[*a,b] b > 1{4[a]
b has to choose one of the 4 areas already taken by a
+ has to touch
M 1/4
R 1,2,1,3,1,5
E |a,b,c|
P *a+b+c
_ has not to touch
A 1/9b
R 2,2,3,1
E a,b,c,d ≈
P
a_b_c_d
a A9[2+2+3+1]
b A9[2+2+3+1]
c A9[2+2+3+1]
d A9[2+2+3+1]
≠ not
M 1/4
R 2,2,3,1
E |a,b|
P
A1 [≠*a]
A2 [≠*a]
A3 [≠*a]
A4 [≠*a]
b M1 *2, M2 *2, M3 *3, M4 *1
V vertical
M 1/9
R 2,2,3,1
E a
P a > 3{1/9 [*a]+[*a]+[*a] V
H horizontal
M 1/9
R 2,2,3,1
E a
P a > 3{1/9 [*a]+[*a]+[*a] H
F flexibel
M 1/9
R 2,2,3,1
E a
P a > 3{1/9 [*a]+[*a]+[*a] F
ⁿ ordinal number
A
A¹ 1/4
A² 1/9
R 2,2,3,1 & 1,2,1,3,1,5
E a
P
a A¹ A3 [*2,2,3,1]
a A² A8 [*1,2,1,3,1,5]
M 1/4
R 1,2,1,3,1,5
E a¹ a²
P
a¹ M1 [*1,2,1,3,1,5]
a² (2\a¹) M4 [*1,2,1,3,1,5]
L line
A 1/144 (L 1-12)
E a
P
L1 A2 [(1)]
L2 A1 [(3)]
L3 A6 [(2)]
L12 A11 [(2)]
{ n } polygon with n edges
A 1/4, 1/9, 1/16
E a,b,c
P
a{3} A1/4
b{4} A1/9
c{5} A1/16
æ all
M 1/4 & 1/9
E a
P a(-)+*æ13M
Comments
Post a Comment